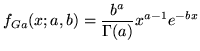

Next: MCMC
Up: Appendix
Previous: Appendix
 has a two-parameter gamma distribution, denoted by
has a two-parameter gamma distribution, denoted by 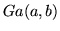 ,
with parameters
,
with parameters 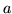 and
and 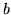 , if its density is given by:
, if its density is given by:
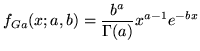 |
(26) |
where 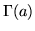 is the Gamma function. Note, that a gamma
distribution has
is the Gamma function. Note, that a gamma
distribution has 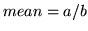 and
and
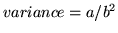 . The mode of the
Gamma distribution is given by:
. The mode of the
Gamma distribution is given by:
 |
(27) |